The dilemma between radiative forcing and the amount of radiation on the Earth's surface
- Antero Ollila

- Sep 28, 2023
- 7 min read
Updated: Nov 5, 2023
Objectives of writing
In this blog, I discuss the issues originally raised by TWTW in its bulletin of 9/16/2023, quoting challenges posed by physicist Howard Hayden in the September 2023 issue of "The Energy Advocate," ref. 1. Hayden challenged climate scientists (specifically those who support the IPCC science) to present their solutions to the following situation:
• Select any SSP. (SSP is the IPCC scenario designation).
• Choose a time in the future. At any time 20 or longer in the future.
• Use the results of that SSP supercomputer code, and present the energy balance calculated using the values of the scenario.
• You must present a numerical value that is missing from the energy balance charts, i.e. the value of the greenhouse effect G.
• You need to show how to calculate the magnitude G of the greenhouse effect from radiative forcing (RF).
Hayden gives a wager of $1,000 to whoever can answer the questions thus asked. I accept the challenge. I know that using the IPCC scientific figures will not match the energy balance figures, but I will present the calculations and figures that otherwise meet the challenges that have been given.
Hayden illustrates the problem by pointing out the figures in the AR6 report for 2019. According to Hayden, from 1750 to 2019, radiative forcing due to anthropogenic causes was 2.72 W/m2 and the resulting temperature change was 1.36 °C. According to figures Fig. 7.6 and 7.7 in AR6, the total forcing was 2.70 W/m2 (the proportion of the sun -0.02 W/m2) and the temperature change was 1.27 °C. According to the law of S-B, an increase in surface temperature of 1.27 °C causes an additional 7.1 W/m2 of radiation from the Earth's surface, while the change in radiative forcing caused by the temperature increase has been only 2.72 W/m2. How can you explain this dilemma?
Global energy balance
First, I present an energy balance picture that also shows the magnitude of the greenhouse effect G, Fig. 1.
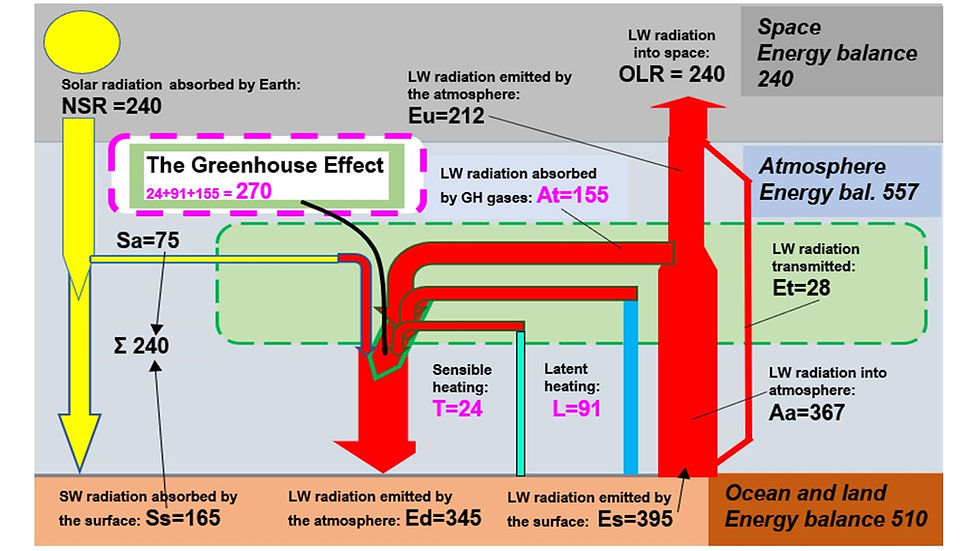
Figure 1. The Earth's energy balance including the magnitude of the greenhouse effect (G=At) as defined by the IPCC. The amount of solar radiation received by the Earth is presented only in net energy values. The figures differ slightly from the values of the IPCC's energy balance but are within the margins of error.
Fig. 1 shows that the actual greenhouse effect is 270 W/m2, which comes from the atmosphere as the sum of three energy fluxes: longwave radiation absorption of 155 W/m2, latent heat 91 W/m2 and hot air upwelling 24 W/m2. These three energy fluxes heat the atmosphere, from where they radiate their energy back to the Earth's surface in the form of infrared radiation Ed. The total radiation received by the Earth's surface SRF=510 W/m2 cannot be explained without this phenomenon as the actual magnitude of the greenhouse effect.
Simple climate model with iteration technology
In my analysis, I use scenario SSP3-7.0, which according to the IPCC means a radiative forcing of 7.0 W/m2 and has a global warming potential of 3.2–3.3 °C. Since I do not have access to GCM models, I use the iteration model I developed (ref. 2) to simulate the effect of radiative forcing on calculating radiant flux values in the energy balance with sufficient accuracy.
My iteration model is based on two basic principles. As the greenhouse effect intensifies, the Es absorption (At) of infrared radiation emitted by the Earth increases in the atmosphere by dAt (=dG) and causes an OLR deviation of the magnitude RF in infrared radiation going into space. The Earth's energy balance will automatically begin to correct the deviation. Since the OLR is now lower than before (OLR-RF), it means that the Earth's surface temperature begins to rise (more energy enters than exits) so that the amount of radiation emitted by it could eventually return to the original OLR value.
The temperature increase is significantly accelerated by the fact that the atmosphere radiates the increased absorption energy dAt back to the Earth's surface, i.e. the basic principle of the greenhouse effect works by recycling the energy of the greenhouse effect absorbed by the atmosphere. The additional energy received by the Earth's surface increases its temperature, and the Earth's surface emits more and more infrared radiation according to Planck's law. The increased amount of infrared radiation increases the magnitude of the greenhouse effect even further because absorption increases, too. This energy re-circulation continues until the radiation OLR going into space has reached its original value, i.e. the net amount of energy the Earth receives from the sun.
My research justifies how I have calculated linear dependencies on the essential variables of the energy balance, which are in W/m2 for energy radiation and °C for temperatures:
· Hot air upwelling T = -7.277 + 1.9867 * Ts
· Absorption of infrared radiation in the atmosphere At = G = 0.4182 * Aa
· Infrared radiation emitted by the earth's surface into the atmosphere Aa = 0.719 * SRF
· Infrared radiation emitted by the earth's surface into space Et = 0.0558 * SRF
· Total radiation emitted by the earth's surface Es = Aa + Et
· Total radiation received by the earth's surface SRF = Ss + Ed = 165 + Ed
· Infrared radiation from the atmosphere to the earth's surface Ed = Sa + L + T + At = 75 + 91 + T + At
· Absorption of solar radiation in the atmosphere Sa = 75
· Absorption of solar radiation to the earth's surface Ss = 165
· Latent heat L = 91
· Ground surface temperature Ts = -55.6684 + 0.18077 *Es.
Table 1. Iteration results for SSP3-7.0 using RF radiative forcing value of 7.0 W/m2. The last row in the table shows the change between the starting point and the end.

The results of the iteration show that when the radiation going into space OLR has returned to its baseline value of 240 W/m2, atmospheric absorption has increased by 11.48 W/m2, whose ratio to RF value is 1.64. Iterations with different RF values showed that this ratio ranged from 1.58 to 1.65. As a rule of thumb, atmospheric absorption increases by about 1.6 times the RF value.
Based on the iteration, the temperature of the Earth's surface has increased by about 2.1 °C. This value can be compared with the value calculated using a simple climate model, which is
dTs = λ × RF (1),
where dTs is the global mean change in surface temperature (°C), λ is the "climate sensitivity parameter", and RF is radiative forcing, i.e. the net change in radiative forcing dF according to the IPCC nomenclature. According to IPCC report AR6, λ has been 0.47 K/(W/m2), which includes positive feedback of water. Without water feedback, the value of λ is 0,27 K/(W/m2) calculated from the current global energy balance, reference 3. The temperature calculated using equation (1) using λ-value 0,47 K/(W/m2) is 3,3°C, fully corresponding to the temperature calculated using the IPCC GCM models. The corresponding value without positive feedback of water, calculated using equation (1), is 1,9 °C. The result of the iteration model corresponds quite well to the climate process, where there is no positive water feedback.
Summary
How well I succeeded in meeting the challenges presented by Hayden:
• Select any SSP. I used SSP3-7.0
• Choose a time in the future. At any time 20 or longer in the future. I chose 2100, which I iterated using eight iteration steps.
• Use the results of that SSP supercomputer code, and present the energy balance calculated using the values of the scenario. I used an iteration model.
• You must present a numerical value that is missing from the energy balance charts, i.e. the value of the greenhouse effect G. I have shown the energy balance in Fig. 1, which shows the IPCC G value of 155 W/m2 and the actual greenhouse effect of 270 W/m2.
• You must demonstrate how G is calculated from radiative forcing (RF). The rule of thumb for an increase of G is dG = 1.6 *RF.
My iteration model illustrates how the greenhouse effect works and why the increase in the greenhouse effect is not the same as the increase in radiative forcing. My results show that IPCC climate models give too large a temperature increase based on RF value due to positive feedback of water that is not present in the atmosphere. The Earth's energy balance and my iteration model show this point as well. This blog does not discuss the calculation of RF, as Hayden did not require; It is a story of its own.
Why climate researchers are quiet about the greenhouse effect (GHE) specification?
Climate scientists and the IPCC are very silent about the specification of the GHE. The philosopher Wittgenstein has formulated the reason for this: Whereof one cannot speak, thereof must be silent.
One good reason to be silent is the fact, that the GHE specification is “IPCC made” from the very first Assessment Report and there is no reference to any published research publications, which is one of the basic principles of the IPCC: we do not make our own research, we only refer and select only the best-published research results. In the AR5 the IPCC wrote that “The downward directed component of this LWR adds heat to the lower layers of the atmosphere and the Earth’s surface (greenhouse effect).” In my research paper published in 2019 (ref. 4), I remarked that the absorption effect is about 155 W/m2, and the downward component is about 345 W/m3, which means that this specification is against the physical laws.
How to be, the IPCC changed the GHE specification in AR6 in 2021 hiding it in the Glossary. Two essential points are that 1) “the net amount emitted to space is normally less than would have been emitted in the absence of these absorbers”; 2) “…anthropogenic emissions contribute to an instantaneous radiative forcing. Surface temperature and troposphere warm in response to this forcing”. This definition avoids saying that there is LW radiation downwards from the atmosphere, which warms up the surface. This is the essential quality of the GHE. I guess that the reason is that the IPCC avoids defining the magnitude of actual LW radiation flux warming up the surface since it is not the same as the absorption of LW radiation caused by GH gases and clouds.
References:
Viite 1. The Energy Advocate. http://www.sepp.org/science_papers/2802.pdf
Dr. Antero OIlila




























Comments